SWIM: About Pages

The word radar is actually an acronym, RADAR, which stands for RAdio Detection And Ranging. Radar systems work by transmitting a radio signal and then measuring how much of that signal bounces back to the receiver and how long it took for that signal to return. When the transmitted signal encounters a contrast in the material that the signal is traveling through, some of the signal is reflected back to the receiver (like light being reflected off of a mirror), some of the signal is scattered away from the receiver, and some of the signal will penetrate through the interface (like seeing through a glass window) where it may be reflected back by another interface further away. The further away the object/interface is that the radio signal reflects off of, the longer it will take the signal to return to the transmitter. Because radio waves are a subset of the electromagnetic spectrum (but at longer wavelengths than the visible light we see with our eyes), they travel at the speed of light (3x108 m/s) through a vacuum (no atoms present). Traveling through different materials causes the radio wave to change and slow down by different amounts, which depends on how easily that material becomes electrically polarized by the incoming electromagnetic radio wave.

The main physical property used to describe the material’s response is called the dielectric constant, or permittivity, and is related to the index of refraction of the material. This property is a complex value, where the real component of the permittivity [“real (part of the relative) dielectric constant”] is related to how much the radar wave is slowed down by traveling through that material compared to the speed of light in a vacuum, and the imaginary component of the permittivity (“dielectric loss”) is related to how much of the signal’s energy is dissipated.

The real part of the dielectric constant ranges from 1 in free space (a vacuum; thus, the speed of the EM wave is not slowed down at all) to 80 or higher for very polar materials like liquid water. Pure water ice has a dielectric constant (real component) of ~3.15 (Matsuoka et al., 1997). Bulk measurements of dense basalts yield dielectric constants in the range of 7.5–9 (e.g., Rust et al., 1999; Campbell and Ulrichs, 1969). The addition of porosity to lithic/rocky materials lowers the real part of the dielectric constant, to the point that very low-density ash can have a similar dielectric constant as ice. Dielectric responses are temperature- and frequency-dependent, and how a mixture (for example, pore space in rock or dust in ice) responds dielectrically depends on a variety of factors, including the bulk properties of both host and inclusion materials, and the shape and orientation of the inclusions (Ulaby et al., 1986). The properties of the medium that the radar signal travels through also affects the attenuation of the radar signal (dielectric loss); pore space, carbon dioxide ice, and water ice are relatively loss-less while lithic materials and liquid water dissipate a lot of the radar power.

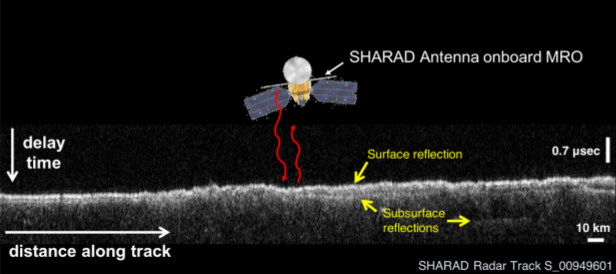
The SHARAD (Shallow Radar) system onboard the Mars Reconnaissance Orbiter is a ground-penetrating radar that transmits a signal with a frequency band of 15 to 25 MHz, corresponding to a maximum wavelength in free space of 15 meters (Seu et al., 2007). The data returned by the instrument are in the form of “radargrams”, which display the power returned (represented by pixel brightness) at various time delays (y-axis) along the track of the spacecraft travel (x-axis) (Figure 1). When the radar wave encounters a contrast in the material properties (e.g., atmosphere to surface or layering in the subsurface), the radar wave can be reflected back, causing an increase in power returned at that delay time (see below). For cases where the depth to a subsurface reflector can be estimated, the thickness of the unit can be combined with the time delay measured with SHARAD to constrain the real part of the dielectric constant of the material through which the radar signal has traveled.

We use a number of techniques for estimating the depth to the subsurface reflector, appropriate to the type of geologic unit we are investigating as well as the availability of topographic constraints.

• In the case of a surface mantle, we attempt to find the edges of the mantle, where the basement geology can be assumed to be continuous with the subsurface reflector observed in SHARAD. When the edge can be identified on either side of the observed mantle, a sloped line is interpolated between the basement elevation at the mantle edges, producing the estimate of reflector depth that can be used to estimate the dielectric constant.
• In the case of a lobate debris apron or other geologic unit abutting the edge of a scarp, only one edge exhibits a boundary where the elevation of the underlying bedrock geology can be constrained. In these cases we either prescribe the nearest bedrock elevation (where it can be reasonably presumed to be flat) or we fit a linear regression to a manually selected section of bedrock and extend that linear interpolation underneath the feature (where slope is observed; manual selection is used to reduce error from e.g. hills and craters and to find the most representative slope).
• In special cases we also use point estimates of the depth to the reflector, from e.g. layer exposures in terraced craters and fossae.